

The compounds with the larger lattice energy are Since the lattice energy is negative in the Born-Haber cycle, this would lead to a more exothermic reaction.Ĥ. 4008 kJ/mol both ions in MgO have twice the charge of the ions in LiF the bond length is very similar and both have the same structure a quadrupling of the energy is expected based on the equation for lattice energyĥ. An equation is developed for calculating lattice energies, using the most readily available experimental data the results are at least as reliable as those. The smaller the radius of the anion, the shorter the interionic distance and the greater the lattice energy would be.In the Born-Haber cycle, the more negative the electron affinity, the more exothermic the overall reaction. A higher electron affinity is more negative. The lattice energy of an ionic compound is the energy change when one mole of ionicsolid is separated into its gaseous ions.The lower it is, the more exothermic the reaction will be. Instead of solving the NavierStokes equations directly, a fluid density on a lattice is simulated with streaming. As in part (b), the bond energy is a positive energy. Lattice Boltzmann methods (LBM), originated from the lattice gas automata (LGA) method (Hardy- Pomeau -Pazzis and Frisch - Hasslacher - Pomeau models), is a class of computational fluid dynamics (CFD) methods for fluid simulation.
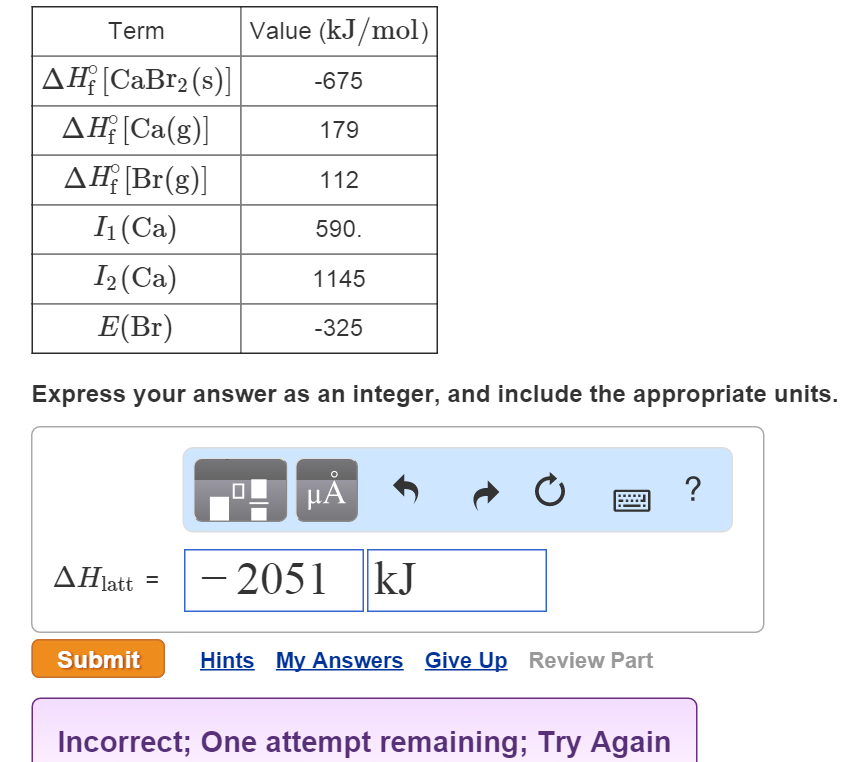
This would make the reaction more exothermic, as a smaller positive value is “more exothermic.” A lower ionization energy is a lower positive energy in the Born-Haber cycle.Since the lattice energy is negative in the Born-Haber cycle, this would lead to a more exothermic reaction. The smaller the radius of the cation, the shorter the interionic distance and the greater the lattice energy would be.Recall that the more negative the overall value, the more exothermic the reaction is. In each case, think about how it would affect the Born-Haber cycle. Along with such, we need to understand that the energy needed to separate 1 mole of a solid crystal into gaseous ions is called lattice dissociation enthalpies. The answer is (d), which requires about 740 kJ/mol.ģ. U may be calculated from the Born-Haber cycle. The lattice energy, U, is the energy required to convert the solid into separate ions. We begin with the elements in their most common states, Cs( s) and F 2( g). The Born-Haber cycle shows the relative energies of each step involved in the formation of an ionic solid from the necessary elements in their reference states.


 0 kommentar(er)
0 kommentar(er)
